This section describes a general theorem for additive measurement in Fishburn (1992b) and applies it to the closed-interval representation
![]()
where ![]() and
and ![]() .
A sample of other approaches to interval and more general threshold
representations is provided in Doignon et al. (1986), Chateauneuf (1987),
Nakamura (1988), Suppes et al. (1989, Chapter 16),
Beja and Gilboa (1992),
Abbas and Vincke (1993),
Bogart and Trenk (1994), Abbas (1995) and Mitas (1995).
.
A sample of other approaches to interval and more general threshold
representations is provided in Doignon et al. (1986), Chateauneuf (1987),
Nakamura (1988), Suppes et al. (1989, Chapter 16),
Beja and Gilboa (1992),
Abbas and Vincke (1993),
Bogart and Trenk (1994), Abbas (1995) and Mitas (1995).
Our theorem is a linear separation theorem for arbitrary systems of
linear inequalities that have finite numbers of terms.
We begin with a nonempty set Y, and let V denote the vector
space of all ![]() for which
for which
![]() is finite. We define
is finite. We define ![]() and v + v' for real
and v + v' for real ![]() and
and ![]() by
by
![]()
The representation for the theorem consists of distinguished subsets A
and B of V and a mapping ![]() for which
for which
where ![]() .
We say that (A,B) is solvable if there exists a
.
We say that (A,B) is solvable if there exists a ![]() that satisfies linear system (10).
We will state a condition on (A,B) that is necessary and
sufficient
for solvability.
It is assumed, with no loss of generality, that the zero function 0
of V is in A and that B is not empty.
that satisfies linear system (10).
We will state a condition on (A,B) that is necessary and
sufficient
for solvability.
It is assumed, with no loss of generality, that the zero function 0
of V is in A and that B is not empty.
A few other definitions are needed.
For ![]() ,
,
![]() .
A subset K of V is a convex cone if it is nonempty,
closed under convex combinations, and contains
.
A subset K of V is a convex cone if it is nonempty,
closed under convex combinations, and contains ![]() whenever
whenever ![]() and
and ![]() .
A convex cone K is without origin if
.
A convex cone K is without origin if ![]() .
The convex cone generated by nonempty
.
The convex cone generated by nonempty ![]() is denoted
by
is denoted
by ![]() , so
, so
![]()
Finally, we say that nonempty ![]() is Archimedean
if for all
is Archimedean
if for all ![]() ,
, ![]() for some
for some ![]() .
.
Our separation theorem says that (A,B) is solvable
if and only if ![]() is included in some Archimedean
convex cone without origin in V.
Given (10), necessity of the condition on
is included in some Archimedean
convex cone without origin in V.
Given (10), necessity of the condition on ![]() is shown by extending
is shown by extending
![]() linearly to all of V by
linearly to all of V by ![]() and observing that
and observing that ![]() is an Archimedean convex cone
without origin that includes
is an Archimedean convex cone
without origin that includes ![]() .
The sufficiency proof is based on a standard separation theorem
discussed, for example, in Kelley and Namioka (1963) and Klee (1969).
.
The sufficiency proof is based on a standard separation theorem
discussed, for example, in Kelley and Namioka (1963) and Klee (1969).
To apply the theorem to the opening representation of this section,
let X' be a disjoint copy of X with ![]() corresponding to
corresponding to ![]() , and let
, and let ![]() .
The opening representation can then be rewritten as
.
The opening representation can then be rewritten as
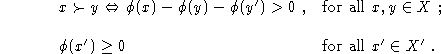
Sets A and B for application of the separation theorem are
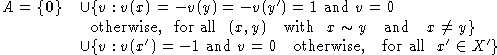
![]()
Suppose ![]() is included in an Archimedean convex cone without origin.
Let
is included in an Archimedean convex cone without origin.
Let ![]() satisfy (10).
Then, when
satisfy (10).
Then, when ![]() and
and ![]() ,
,
![]() and
and
![]() , or
, or ![]() and
and
![]() ;
for
;
for ![]() ,
,
![]() ,
or
,
or ![]() ;
when
;
when ![]() ,
,
![]() , or
, or
![]() .
.